
engineering & technology publications
ISSN 1759-3433
PROCEEDINGS OF THE EIGHTH INTERNATIONAL CONFERENCE ON CIVIL AND STRUCTURAL ENGINEERING COMPUTING
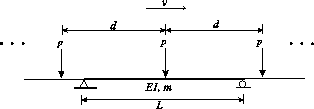
Impact Envelope Formula of Simple Beams due to High Speed Trains
+Department of Architecture and Building Technology, Tamkang University, Taipei, Taiwan
*Department of Civil Engineering, National Taiwan University, Taipei, Taiwan
where
On the other hand, whenever the following condition is met, that is,
![]() , the dynamic response for cancellation
, the dynamic response for cancellation
![]() in equation (33.1) becomes
in equation (33.1) becomes
where the subscript
In engineering practice, the damping effect of structures should be included.
In this study, the damping ratio of simple beams is assumed to be small, i.e.,
with
![]() , and the operation speed range is assumed to be
, and the operation speed range is assumed to be
![]() )
for moving vehicles, which implies that terms involving
)
for moving vehicles, which implies that terms involving ![]() and
and
![]() , can be
neglected. Under such a circumstance, the resonant response of the simple beam
subjected to a sequence of moving loads can be obtained as:
, can be
neglected. Under such a circumstance, the resonant response of the simple beam
subjected to a sequence of moving loads can be obtained as:
where
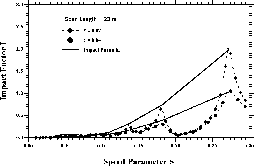
For different damping ratios, the envelope impact formula of equation (33.4) has been plotted in Figure 33.2. As can be seen, for the case of light damping, the envelope impact formula has a trend in good consistency with the
purchase the full-text of this paper (price £20)
go to the previous paper
go to the next paper
return to the table of contents
return to the book description
purchase this book (price £122 +P&P)
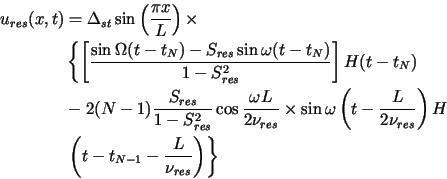
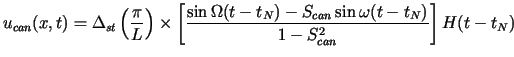
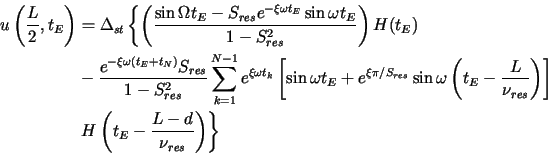
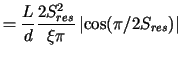 for resonance
for resonance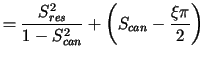 for resonance
for resonance